Two-Block Problems

Two blocks connected by a massless string slide down an inclined plane having an angle of inclination of  $37°.$  The masses of the two blocks are  $M_1=4kg$  and  $M_2=2kg$  respectively and the coefficients of friction of  $M_1$ and  $M_2$ with the inclined plane are 0.75 and 0.25 respectively. Assuming the string to the taut, find the tension in the string.  $\left(\sin 37°=0.6,\cos 37°=0.8\right)$

Two blocks connected by a massless string slide down an inclined plane having an angle of inclination of $37°.$ The masses of the two blocks are $M_1=4 \mathrm{kg}$ and $M_2=2 \mathrm{kg}$ respectively and the coefficients of friction of $M_1$ and $M_2$ with the inclined plane are $0.75$ and $0.25$ respectively. Assuming the string to be taut, find the common acceleration of two masses. $\left(\sin 37°=0.6, \cos 37°=0.8\right)$

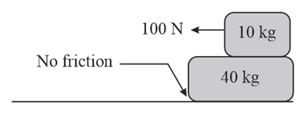
In the arrangement coefficient of friction between the two blocks is $\mu =\frac{1}{3}$. The force of friction acting between the two blocks is: $\left(g=10 \mathrm{m} {\mathrm{s}}^{-2}\right)$

A man sitting in a train in motion is facing the engine. He tosses a coin up, the coin falls behind him. The train is moving

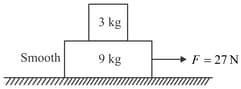
A single horizontal force F is applied to a block of mass $M_1$, which is in contact with another block of mass $M_2$(Given figure). If the surfaces are frictionless, the force between the blocks is

Two blocks of masses $M=5 \mathrm{kg}$ and $m=3 \mathrm{kg}$ are placed on a horizontal surface as shown in fig. The coefficient of friction between the blocks is $0.5$ and that between the block $M$ and the horizontal surface is $0.7$. What is the maximum horizontal force $F$ that can be applied to block $M$ so that the two blocks move without slipping ? Take $g=10 \mathrm{m}/{\mathrm{s}}^2$

Blocks $A$ and $B$ are arranged as shown in the figure. The pulley is frictionless. The mass of $A$ is $10 \mathrm{kg}$. The coefficient of friction between the block $A$ and the horizontal surface is $0.20$. The minimum mass of $B$, to start the motion, will be

In the arrangement shown in the figure, if the blocks of masses $m$ and $2 \mathrm{m}$ are released from the state of rest, tension in the string is ($\mu =$ coefficient of friction, string is massless and inextensible, pulley is frictionless)

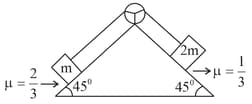
In the situation shown, the reading of the spring balance is $\left(g=10 \mathrm{m} {\mathrm{s}}^{-2}\right)$

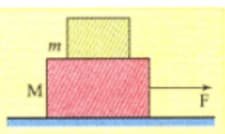
If $F$ force is applied to $10 \mathrm{kg}$ block as shown in diagram then maximum value of $F$ so that there is no relative motion between the blocks.

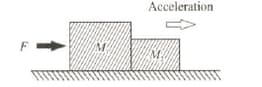
Calculate the accelerations of the blocks $A$ and $B:-$

A force of $0.5 \mathrm{N}$ is applied on the upper block as shown in figure. The coefficient of static friction between the two blocks is $0.1$ and that between the lower block and the surface is zero. The work done by the lower block on the upper block for a displacement of $3 \mathrm{m}$ of the upper block is :-

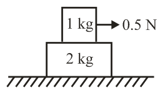
In friction is present between $A \text{\&} B$ only and $F>$ limiting friction between $A\text{ \&} B$. Find acceleration of $A \text{\&} B\mathit{.}$

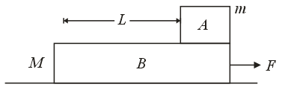
In the given arrangement of a doubly inclined plane two blocks of masses $M$ and $m$ are placed. The blocks are connected by a light string passing over an ideal pulley as shown. The coefficient of friction between the surface of the plane and the blocks is $0.25$. The value of $m$, for which $M=10 \mathrm{kg}$ will move down with an acceleration of $2 \mathrm{m} {\mathrm{s}}^{-2}$, is : (take $g=10 \mathrm{m} {\mathrm{s}}^{-2}$ and $\tan 37°=\frac{3}{4}$)

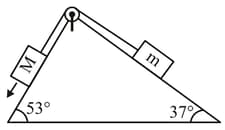
A block of mass $m$ sits on the top of a block of mass $2m$ which sits on a table. The coefficient of kinetic friction between all surface is $\mu = 1$ . A massless string is connected to each mass and wraps halfway around a massless pulley, as shown. Assume that you pull on the pulley with a force of $6mg$. The acceleration of your hand is -

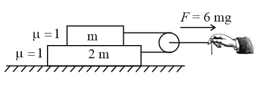
Two blocks of masses $\mathrm{m}$ and $2\mathrm{m}$ are placed one over the other as shown in the figure. The coefficient of friction between $\mathrm{m}$ and $2\mathrm{m}$ is $\mathrm{\mu }$ and between $2\mathrm{m}$ and ground is $\frac{\mathrm{\mu }}{3}$. If a horizontal force $\mathrm{F}$ is applied on the upper block and $\mathrm{T}$ is the tension developed in the string, then choose the incorrect alternative.

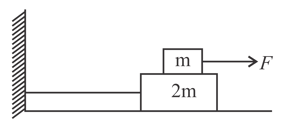
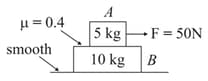
In the figure, $m_A=2 \mathrm{kg}$ and $m_B=4 \mathrm{kg}$. For what minimum value of force $F$, $\mathrm{A}$ starts slipping over $\mathrm{B}$ $\left(g=10 \mathrm{m} {\mathrm{s}}^{-2}\right)$

Two blocks $\mathrm{A}$ and $\mathrm{B}$ are placed one over the other on a smooth horizontal surface. The maximum horizontal force that can be applied on the lower block $\mathrm{A},$ so that $\mathrm{A}$ and $\mathrm{B}$ move without separation is $49\mathrm{ N}$. The coefficient of friction between $\mathrm{A}$ and $\mathrm{B}$ is ( take $\mathrm{g}=9.8 \mathrm{m} {\mathrm{s}}^{-2}$ )

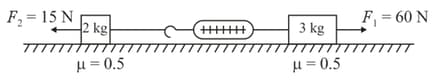
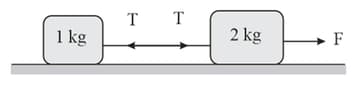
Two blocks masses 1 kg and 2 kg rest on a smooth horizontal table. When the 2 kg block is pulled by a certain force $F$, the tension $T$ in the string is 1.5 N. The value of $F$ is

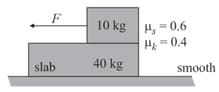
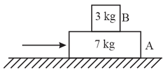
A $40 \mathrm{kg}$ slab rests on a frictionless floor. A $10 \mathrm{kg}$ block rests on top of the slab. The static coefficient of friction between the block and the slab is $0.60$ while the kinetic coefficient of friction is $0.40$. The $10 \mathrm{kg}$ block is acted upon by a horizontal force of $100 \mathrm{N}$. If $g=9.8\mathrm{ }\mathrm{m}{\mathrm{s}}^{-2}$, the resulting acceleration of the slab will be